The study builds on the results of an article from James Coleman entitled 'Norm-Generating Structures'. In this article Coleman considers whether cooperative behavior establishes itself through evolution as the norm in a social group when group members encounter foreigners occasionally. Since people cooperate in closed social groups the question that Coleman asks is: in what ways cooperation with foreigners depends on the degree of social closure? In the article mentioned above Coleman is only able to outline the answer to this question. The goal of this contribution is to broaden Coleman's model and completely answer the question, whereby it will become clear that Coleman's results only represent a special case. In general the evolution of cooperation with foreigners is unpredictable, but a low level of xenophobia can be established through evolution under a broad range of circumstances.

Keywords:

Evolution Of Cooperation; Social Closure; Xenophobia

Introduction

1.1

This study builds on the results of an article from James Coleman entitled "Norm-Generating Structures," which was published in 1992 in an anthology entitled "Limits of Rationality" (Coleman 1992, Coleman 1986^[1]). In this article Coleman considers whether cooperative behaviour establishes itself through evolution as the norm in a social group when group members encounter foreigners occasionally. An encounter with a foreigner is an uncertain situation. But when cooperation is typical in these situations this behaviour can be referred to as "the norm of cooperation"^[2].

1.2

"Foreigners" are people whose behaviour is unfamiliar as well as people with whom one cannot communicate. Therefore cooperative behaviour towards foreigners can be better understood if we construct a model that is based on the one-round prisoner's dilemma game. Robert Axelrod (1984, Hoffmann 2000) proved that cooperative behaviour is advantageous for two people who find themselves in a prisoner's dilemma situation repeatedly. This is because opponents get to know one another after a few encounters and can coordinate their behaviour. Therefore cooperative behaviour is also advantageous in a closed social group when pairs of people are confronted with the prisoner's dilemma situation often enough^[3], and in such cases cooperation spreads through evolution.

1.3

But how do individuals behave when they are in a social group that is not closed and occasionally encounter foreigners who they are not likely to encounter again in the future? When the number of remaining encounters is finite, and players know when the game will terminate, it makes more sense to collect a defection payoff early on^[4]. In other words, it is better not to cooperate with foreigners.

1.4

This problem has been dealt with in a number of ways. One possible approach is to introduce a third possible manner of behaviour: one removes himself from the confrontation with a foreigner by "refusing [to participate in] the PD-Game that has been offered". The inclusion of this possibility adds an additional, realizable characteristic, the willingness to play, which people can notice and which leads to the fragmentation of the society into two subgroups - a cooperating and a defecting one (Orbell, Zeng and Mulford 1996, Hausken 2000). In addition, one can divide the players into subgroups by differentiating spatially between "neighbours" and others who are distinguished by the frequency of their interactions. Macy and Skvoretz (1998) use this approach and even go on to include further individual characteristics, such as whether or not people greet each other. In this manner, a very complex system of possible developments is fashioned. This and similar systems have often been simulated by means of the techniques used for genetic algorithms - techniques which are used to change and test strategies by means of mutations. The stable end conditions that this approach produces are difficult to understand as products of rational behaviour. A third possibility is to increase the length of the interaction sequences with foreigners (Vogt 2000) so that, for example, people remember the last three moves they made. Additionally, noise - the possibility of 'false' memory - can be introduced as well. To come to terms with the resulting dynamic, Lomborg (1996) also employed genetic algorithms. Since the mix of strategies that characterizes a variety of stable and low-vulnerable populations is only to be understood as a plead for pluralism (Lomborg 1996:301), it cannot be used to substantiate the "norm" of co-operation. These modifications change the nature of the game to such an extent that it might cease to be a PD-game.

1.5

Coleman's basic approach is less complex and concentrates on the first move people make when they encounter "foreigners" in iterated PD-games. When co-operation is the norm, this dictates that people will cooperate with a foreigner during the first PD-Situation. Focus is placed on the stability of cooperation in the first game, in other words, the decision to "cooperate" in the first move of a strategy. Since people cooperate in closed social groups, the question that Coleman asks is - where does the border lie between cooperation (within closed social groups) and defection (amongst foreigners) that allows cooperative behaviour to establish itself through evolution in more or less closed social groups? More precisely, he poses the following two questions:

1. How does the degree of cooperation relate to the degree of social closure?
2. 1. Will cooperation with "foreigners" spread?
   2. And in what ways is cooperation with foreigners dependent on the degree of social closure?

1.6

In the article mentioned above Coleman is able to answer the first question partially but he is only able to outline the answer to the second question. The goal of this contribution is to broaden Coleman's model and completely answer both questions^[5]. In the next section (section 2) I will explain how Coleman incorporates the questions listed above into the model of a theoretical game - a model that Coleman uses as a framework to answer both questions. In section 3 I will answer the 1st question and present Coleman's results more precisely. In section 4 I will answer the second question, whereby it will become clear that Coleman's results only represent a special case. In general the evolution of cooperation with foreigners is unpredictable.

The Model

2.1

Coleman uses a model he calls "Strategic Actors in Interaction" to analyse the decision to cooperate in a social group. I will now present the details of this model^[6]. Coleman's model is an attempt to describe both questions by using a minimal number of assumptions. But this attempt alone leads to complex results.

Interactions

2.2

The social situations in which cooperation or defection can take place are represented by a prisoner's dilemma-situation. In each case two people who do not have the ability to communicate encounter one another and must decide for or against cooperation simultaneously. Therefore these sequential interactions are iterated prisoner's-dilemmas^[7].

Social Structure

2.3

Coleman's model is based on a social group with a fixed population of size N (N > 2). Interactions can only take place within this group. When N is small only a few people know each other and group members often have the same interaction partners. But when N is large group members know many people even though they seldom interact with the same people more than once^[8]. In this model the group size N is therefore one of two indicators for the degree of "closure" in a social structure.

2.4

In Coleman's original paper a community consists of a total of n separated social groups of size N and the simulation took place separately in each of the groups. Thus n represents the number of repetitions of the same simulation to achieve stable simulation results with little variance.

"Foreigners"

2.5

Interactions within the groups take place between two randomly selected people at a time. Because of the size of the groups people will meet others with whom they have had various degrees of contact:

• the person is "foreign": = the person has never been encountered,
• the person is a "new acquaintance": = the person has already been encountered once,
• the person is an "old acquaintance": = the person has already been encountered more than once.

According to Coleman, further levels of repeated contact will not be considered here because of the use of a modified TFT-strategy (see below, paragraph 2.9) which only has a length of 2.

Memory Length

2.6

In this model people are equipped with a limited ability to recall which people they have met and which cooperation decisions they have made. If, for example, the memory length is 4, they can remember the last 4 people they have met and the moves that were exchanged. Therefore the memory length k determines the frequency with which people encounter "foreigners". If k is short and the group is large, people will seldom meet someone they recognize. But if k is long, much longer than the size of the group for example, people will seldom meet foreigners. Therefore memory length is the second indicator of the degree of social closure^[9].

Social Closure

2.7

The combination of group size and memory length determines the degree of social closure in a social structure. This combination also effects each person's degree of forgetfulness over a period of time^[10]. Given group size N and memory length k, the probability that a person will meet a "foreigner" is affected in the following ways:

• The larger the group, the more often people will meet foreigners.
• The shorter the memory length, the more often people will meet foreigners.

2.8

For clarity's sake, social closure is a term that, thanks to Weber, is used to refer to the degree to which social classes are isolated from one another (Weber 1976:177f.). The frequency of interactions with persons who are not members of one's own class is therefore a measure of the degree of closure within a class. The model does not account for this definition directly, as it cannot identify a fixed number of persons who belong to a class or group and another set of persons who are "foreigners". Instead, every person may become "foreign" to another person who does not remember his or her last encounter. But regarding cooperation with unknown persons, it is equivalent if the person who I meet for the first time is not a member of my class or if he/she is unknown because of my limited memory. Additionally, the model fits Weber's most often cited definition quite well, according to which "a social class consists of class positions between which an exchange of persons .. is easily possible" (Weber 1976:177). Weber's definition is fitting if one equates an easy exchange of persons with the frequent interaction of persons.

Strategy

2.9

In this model people behave as it is assumed they will in a prisoner's dilemma situation (Rapoport and Chammah 1965). They interpret a hostile move as a "reaction" to their own prior behaviour. In the course of a series of meetings they try to encourage others to cooperate on issues such as punishment and recompense since such a strategy produces the highest payoff. Therefore in the long run they behave as if they were using a tit-for-tat strategy. But this strategy must be modified to fit the conditions of a larger group. Since interactions in a large group can take place between foreigners and between new or old acquaintances, there are seven reactions that are possible when people only consider the opponent's reaction to their own preceding move.

Table 1: Person A's reactions when he/she encounters Person B (C = cooperation, D = defection)
	Person-B is	Person-A's previous move	Person-B reacted with		Person-A's move will be
		n-2	n-1		n
1	a foreigner	-	-	=>	D
2	a new acquaintance	-	C	=>	C
3	a new acquaintance	-	D	=>	D
4	an old acquaintance	C	C	=>	C
5	an old acquaintance	C	D	=>	D
6	an old acquaintance	D	C	=>	C
7	an old acquaintance	D	D	=>	C
(Coleman 1992:Table 7.2)

2.10

In moves 2 through 6 people use the well-known Tit-for-Tat moves - the reactions to cooperation and defection are the same. But in move 1 Person-A tries to exploit foreigners by profiting from a defection. This behaviour will be referred to as "xenophobia," even though the two persons do not belong to different groups (see 2.5 to 2.8. above). Since this behaviour is very important for the consideration of our question we will change our model later so that some people cooperate with foreigners. This behaviour will be referred to as "cooperation". Move 7 leads to cooperation because Person-A interprets Person-B's defection as punishment for his/her own preceding defection. Otherwise, of course, no cooperative behaviour whatsoever would have the chance to develop^[11].

2.11

In this model it is assumed that only one strategy is used, namely the strategy used in the chart above in all moves except the first. The value of this model is not that it allows us to consider the effects produced by various strategies used during long-term conflicts with old acquaintances. Rather its value is that it helps us better analyse the decision to cooperate with foreigners during the first move. It is this decision that allows us to assume that a fixed optimal strategy will also be used during following encounters^[12].

Fixed Strategies

General Xenophobia

3.1

Using these assumptions and the help of a simulation program^[13] we can now determine the answer to the first question. For the time being there are two parameters at play that determine long-term cooperation behaviour, group size and memory length. Coleman obtained the following results:

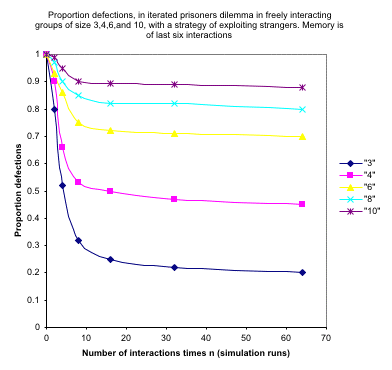
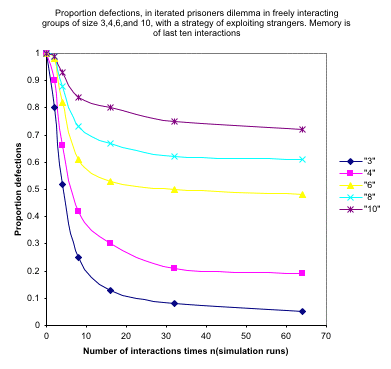
Figure 1. (after Coleman 1992: Figures 7.2, 7.3)

3.2

The variable that Coleman chose to describe the conditions in this social system, the total number of defections, is represented by the vertical axis. This variable shows first of all how cooperative the people in this system are and secondly it is an indicator for the value of the total payoffs that result from cooperation^[14]. The horizontal axis represents the average number of interactions per group participant (70 = on average, 70 interactions per member of each of the n simulation runs).

3.3

Coleman allowed for as many as 70 interactions per group participant. But it is unclear if the value that is reached after 70 interactions remains stable in the long run. Could it be that in groups of every size cooperation no longer takes place when interaction sequences are sufficiently long? When the probability of encountering a "foreigner" is not yet 0 a defection will take place every time a "foreigner" is encountered. But, as already mentioned above, the larger the group size and the smaller the memory length, the larger the probability of meeting a foreigner.

3.4

This means that for every given group size the proportion of defections can be reduced as much as desired by assuming that memory length is much larger than group size. But this is unrealistic; memory depends on rather complex learning processes. Nevertheless, none of these processes might be able to increase memory length arbitrarily. This leads to the following result: according to this model the fact that there is always a certain proportion of defections in large groups is quite normal. The following figure is a generalization of Coleman's partial results and shows group conditions following 1000 interactions and thus the long-term proportions of defections as well (proportions that have already reached their lower limits and cannot be further reduced):

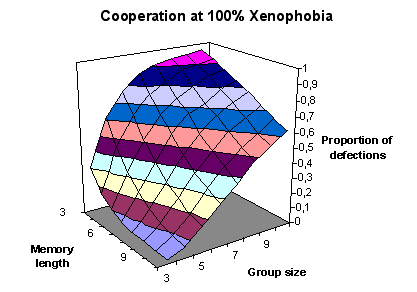
Figure 2. Cooperation (measured by the proportion of defections) at 100 per cent xenophobia - variables: group size and memory length

Partial Xenophobia

3.5

The next parameter that is interesting as regards our question is behaviour towards foreigners, which is established in strategy-move 1. This move demonstrates that foreigners are, in principle, exploited. But such strictly rational behaviour is somewhat implausible. When we assume that not all group members, but only a certain proportion, are xenophobic, then our model is much more variable. Therefore, a third essential parameter is the proportion of "co-operators", namely the proportion of people who cooperate in move 1. For example, when the proportion of co-operators is 50 per cent the following long-term (1000 iterations) image is produced, an image which also includes Coleman's partial results (1992: Figure 7.4):

Figure 3. Cooperation (measured by the proportion of defections) at 50 per cent xenophobia - variables: group size and memory length

3.6

The long-term proportion of defections is approximately half as large on all points of this plane as when there is 100 per cent xenophobia. In terms of theory alone this is easy to understand: Since co-operators never defect when they meet foreigners, the proportion of defections is proportional to the proportion of xenophobic people. Therefore

This proportionality explains the corresponding halving of the graphic.

3.7

When group size is set a lower limit is produced below which the proportion of defections cannot fall. The value of this lower limit depends on memory length and the proportion of xenophobes. In groups of size 10, for example, the following limits result

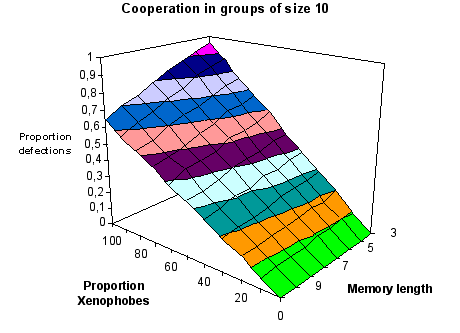
Figure 4. The proportion of defections in groups of size 10 - variables: memory length and the proportion of xenophobes

Taking 3 parameters into consideration allows us to make the following conclusion: There is very little cooperative behaviour in large groups when the majority of group members are not co-operators from the start.

Variable Xenophobia

4.1

The above result is therefore a perfect example of the principle that was the basis for early political theories from, for example, Hobbes (1950 [1651]) or Rousseau (1950 [1756]). According to these theories, a general state of peace cannot be achieved when social interactions occur in a state of nature, but only when supplementary powers, sovereignties, princes or institutions are introduced. This leads us to an interesting question: Does our model allow cooperative behaviour to spread and establish itself in larger groups even if further assumptions are not introduced^[15]?

4.2

In the scenarios considered thus far xenophobic behaviour was treated as a set social element. Thus the fixed and unchanging proportion of xenophobes determined the long-term fate of the social system beforehand. But when one thinks in terms of long-term developmental time spans such rigid and unchanging behaviour towards foreigners is not very plausible. Therefore in the course of a series of interactions strategy changes should be possible that allow xenophobes to become co-operators. In accordance with a rational strategy the payoffs received in the course of a series of interactions must be evaluated before people can decide for or against a change in behaviour. Thus, one allows for a mechanism that is allowed for in other evolutionary games as well - when interaction payoffs are small, a switch is made to a strategy with better prospects. In this case one can only choose between two strategies - to "cooperate" or "defect". In other words, one must choose to change or not change one's first move in the strategy table.

4.3

To calculate payoffs a matrix must be constructed which corresponds to the conditions of the prisoner's dilemma scenario. In this case Coleman chose a payoff matrix that deviates from typical payoff matrices:

	Axelrod			Coleman
	B Coop	B Def		B Coop	B Def
A Coop	3, 3	0, 5	A Coop	0, 0	-2, 1
A Def	5, 0	1, 1	A Def	1, -2	-1, -1

4.4

Coleman ensured that his payoffs correspond to the conditions of the prisoner's-dilemma. Using Coleman's values the long-term average payoffs (i.e. sums divided by the number of interactions) will lie between 0 and -1. People who only cooperate mutually will receive values near 0 and people who only defect mutually will receive values near -1. People who do not exclusively cooperate or defect will receive values that lie between these two extremes. At the very beginning, average payoffs can lie above 0 or below -1. In order to calculate probabilities these payoffs are bounded at 0 resp. -1. Coleman selected his values so well that the inverse of the average payoff value can represent a probability, namely the probability that a strategy change will take place. Because people with payoff values near 0 have done well it is very unlikely that they will change strategies. But people with payoff values near -1 are likely to change their strategies because they have not done well in comparison to others.

4.5

If people change their strategy, their previous experiences ("memory") as well as the previous experiences of the people who they came in contact with cannot be used to make decisions during following interactions. For this reason the rules of the game require that a person who makes a strategy change "dies" and is replaced by a new person who uses the alternative strategy. (This is the technical equivalent of erasing a person's memory along with other people's memories of their interactions with the person whose memory is erased.)

4.6

With this rule in place intuition alone cannot tell us what evolutionary developments will lead to: strategy changes will cause

• on the one hand, an increase in the proportion of "co-operators" because of the better payoffs of this strategy,
• and, on the other hand, an increase in the proportion of "foreigners" (because many people have "died") leads to an increase in the proportion of xenophobes since xenophobes receive higher payoffs when new foreigners cooperate during the first move.

Long-term Cooperation with Foreigners is Unpredictable

4.7

With these new evolutionary conditions in place Coleman obtained the following results^[16]:

Figure 5. (after Coleman 1992: figure 7.5)

4.8

Instead of showing the total proportion of defections, as was previously the case, this time the vertical axis shows the proportion of xenophobes. Coleman also set the memory length at 6 and chose 50 per cent as the starting proportion of xenophobes. In this case the proportion of xenophobes remains stable in smaller groups, but in larger groups under the same conditions the proportion of co-operators is reduced and xenophobia spreads! This result is counter-intuitive^[17]. But, since the graph only shows a small portion of possible developments and since long-term developments still cannot be estimated after 32 interactions (x-axis: 32 = average of 32 interactions in each of the n simulation runs), further results can be obtained with a simulation program.

4.9

Now a fourth parameter comes into play, the number x, which represents the number of interactions that must take place before people take a look at their payoffs and decide for or against a strategy change. The following can be said about the value of x: If x is large in comparison to the memory length the system is already well on its way to the long-term "end-state". In this end-state people are no longer foreign to one another, cooperation is common, and payoffs don't vary much, which means that strategy changes no longer take place. Therefore, so that evolution can have any effect at all, the value x in this system should not be too large.

4.10

On the other hand, if x is small in relation to the total number of people, people will meet foreigners more often than new and old acquaintances in the period of time leading up to a strategy change. Therefore people will change their strategies more frequently since meetings with foreigners often lead to losses.

4.11

A simulation with four parameters makes it somewhat difficult to determine the regularity of effects. But Coleman's parameters provide a base for us to start from - the proportion of xenophobes is 50 per cent and a strategy change is possible after 6 interactions. These parameters support Coleman's results in the long run:

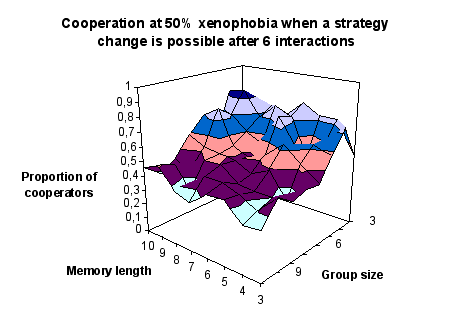
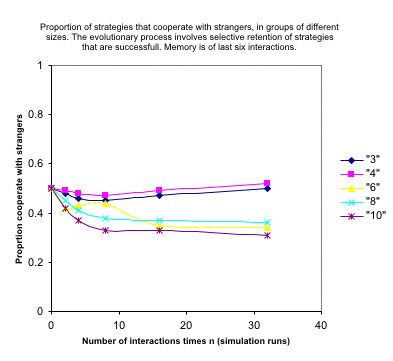
Figure 6. Long-term proportion of co-operators when the starting proportion of xenophobes is 50 per cent and a strategy change is possible after 6 interactions. The values shown at memory length 6 in this graph represent the ending values of the curves in figure 5.

Counterintuitive in this case is that memory length does not appear to play a large role in determining the long-term proportion of co-operators and that the proportion of co-operators is subject to strong chance fluctuations.

4.12

A return to the means of presentation used in the previous graph, namely a consideration of the long-term proportion of defections instead of the proportion of co-operators, produces the following results:

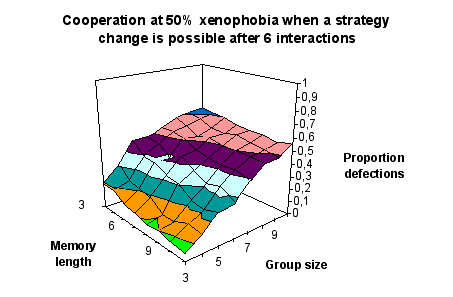
Figure 7. Cooperation (measured by the proportion of defections) when the proportion of xenophobes is 50 per cent and a strategy change is possible after 6 interactions

Here again we see the influence of memory length but only in smaller groups. In larger groups the variable memory length leads to completely counterintuitive results - when an increasing number of interactions are remembered, the proportion of defections climbs and remains above 50 per cent. But chance fluctuations appear to be at work here as well.

4.13

These unusual results can be understood when one follows individual trends in behaviour towards foreigners. The following two trends, for example, reflect the mechanisms that are essential for an understanding of these results:

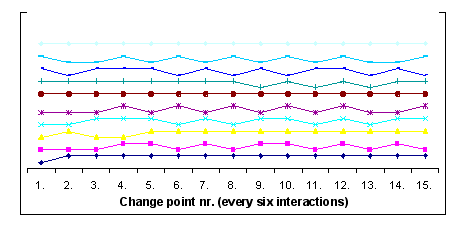
Figure 8. A single run of the changes in behaviour towards foreigners - starting conditions: group size 10, memory length 10, starting proportion of xenophobes 50 per cent, and strategy change possible after every sixth interaction. The strategy selected is indicated in the following manner, low point = cooperate, high point = defect

4.14

At the beginning there are 5 co-operators (represented by the 5 lower paths in Figure 8) and just as many xenophobes (represented by the 5 upper paths). After one round all people have participated in approximately 6 interactions and have reached a given strategy change probability (-payoff/interaction) of 6/8, 1/6, 2/3, 1/6, 3/6, 1/3, 0/4, 4/7, 3/3, 4/10^[18]. The people with absolute values larger than 0.5 will change strategies, as is here the case for the 1^st, 3^rd, 8^th and 9^th person (from below). These people start counting their interactions anew, while the others continue to count where they left off. As chance may have it, the paths do not stabilize at all as time goes on; there are constant strategy changes and in the long run the number of xenophobes fluctuates between 4 and 9.

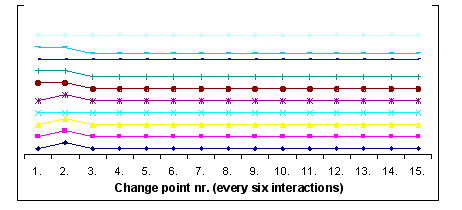
Figure 9. Changes in behaviour towards foreigners during another single run when starting conditions are the same as in figure 8

4.15

The initial conditions in Figure 9 are the same as in Figure 8. But this time things stabilize after the third round; no more strategy changes take place and two xenophobes are retained. Things will remain this way as time goes on. What happened here? In this case, as a result of randomly determined encounters, there are 8 co-operators in round 3 (with respective strategy change probabilities of 2/6, 2/6, 2/4, 7/19, 0/7, 1/5, 0/4, 1/17, 2/9 and 5/18) and because there are only two xenophobes left, occasional encounters with xenophobes cannot lead to significant increases in these values. Thus, after the 8 ^th round the quotients have generally fallen in value: 8/35, 3/28, 3/38, 11/47, 5/32, 5/25, 4/31, 4/40, 3/36, and 8/49. As well, strategy changes have stopped, and the system has stabilized at 2 xenophobes.

4.16

Although the starting conditions are the same, these two figures provide evidence for two very different trends. After taking a look at Figures 8 and 9 one can imagine that these systems could stabilize at various proportions of xenophobes and could also continue to be unstable forever. Therefore we can conclude the following: the end-state that a social system with these starting conditions produces is self-organized^[19] and unpredictable!

4.17

This surprising result explains the large fluctuations in Figures 6 and 7. When things do not stabilize the proportion of xenophobes fluctuates within a broad range and produces an accordingly large proportion of defections because of repeated encounters with foreigners. But if things do stabilize, strategy changes stop and a specific proportion of xenophobes is retained. In this case the proportion of defections returns to exactly the value that was calculated above for this proportion xenophobes, namely the value that is produced when a strategy-change does not take place (section 3.5). But since things stabilize by chance, the proportions of xenophobes in the moment of stabilization vary. Therefore the long-term proportion of defections will vary as well.

4.18

Since it is more likely that conditions will stabilize in smaller groups, the long-term proportions of defections in these groups are similar to the long-term proportions of defections when a strategy change is not possible. But in larger groups the proportions of defections are large, on average, even when the memory length is larger, because stabilization doesn't take place.

4.19

Thus it is clear, for the time being, how Coleman's results can be explained precisely: The possibility of a strategy change leads, self-organized, to a large range of system paths that are characterized by a large variety of long-term xenophobe and defection proportions. For this reason, especially in larger groups, the average proportion of defections is not reduced at all and even reaches values that lie above the values produced when a strategy change is not possible.

Conditions Necessary for long-term Cooperation with Foreigners

4.20

Based on the results presented thus far it seems as though an "optimising rule" cannot be used to induce an evolutionary process so that a small proportion of defections can even be established in larger groups. But as is to be expected when four parameters are at play, there are complex relations in these systems that still need to be considered. For example, it is still not clear how the values x (the number of interactions that must occur before a strategy change is possible) and p₀ (the starting proportion of xenophobes) work together to affect the proportion of defections. For this reason we will now consider a calculation of the long-term proportion of defections when the variable parameters are p₀ and x, while group size is set at 10 and memory length at 6.

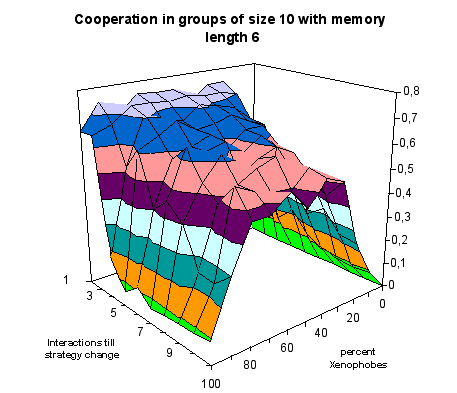
Figure 10. Cooperation in groups of size 10 with memory length 6 - variables: the starting proportion of xenophobes and the number of interactions till a strategy change can take place

4.21

This calculation produces an image similar to that of a narrowing tunnel. The results of Figures 7, 8 and 9, which were calculated by using a starting proportion of 50 per cent xenophobes, lie on the backside of the tunnel. In the previous examples 50 per cent was selected as the starting proportion of xenophobes because it seemed, intuitively, to be a figure that would allow for an improvement in group conditions, namely a reduction in the number of xenophobes. But Figure 10 shows, that in this case a starting proportion of approximately 50 per cent xenophobes leads to the highest level of defection! This proportion of xenophobes is the most inappropriate proportion that Coleman could have chosen. Large as well as small starting proportions of xenophobes (in the last case this seems to make sense intuitively) lead to a reduction in the number of defections. Especially when the starting proportion of xenophobes is large, long-term reductions in the proportions of xenophobes can be achieved, proportions that lie far below the values reached when a strategy change is not possible. In general, this result is the same for all values of x, the number of interactions that must take place before a strategy change can be made. But the larger the number of interactions between strategy changes, the larger the reduction in the proportion of xenophobes.

4.22

How can this be explained? The following two graphs provide an answer:

Figure 11. Changes in behaviour towards foreigners during a single run - starting conditions are the same as in Figure 8 but with a starting proportion of xenophobes 90 per cent, strategy change possible after 6 interactions

The conditions in Figure 11 are the same as in Figures 8 and 9, but in this case the proportion of xenophobes is 90 per cent. At the beginning the high level of xenophobia leads to low payoffs and a dramatic number of people switch to strategies of cooperation. As a result of the mechanism described above, these strategy changes stabilize after just a few more rounds till the xenophobes account for only 40 per cent of the population, which means that there is a 50 per cent reduction in the proportion of xenophobes (from 90 per cent to 40 per cent xenophobes). Therefore the long-term developments in this system tend towards the same proportion of xenophobes that is produced when strategy changes are not possible and the proportion of xenophobes is fixed at 40 per cent.

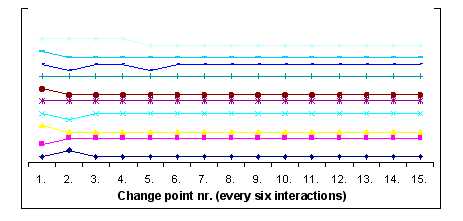
Figure 12. Changes in behaviour towards foreigners during a single run with the same starting conditions as in Figure 8, but in this cast the starting proportion of xenophobes is 80 per cent

4.23

Figure 12 shows the same process as Figure 11 when the starting proportion of xenophobes is 80 per cent. In Figure 12 the stabilization process is slower but after 7 rounds the proportion of xenophobes is also reduced by 50 per cent and the conditions stabilize.

4.24

A large starting proportion of xenophobes will be reduced because xenophobes cannot collect defection profits amongst themselves and switch to strategies of cooperation. But if the proportion of co-operators and defectors is similar, xenophobic behaviour will be retained because of the frequent opportunity of collecting a defection profit.

Results

5.1

The question posed at the start of this paper was if cooperation can be established through evolution in open social structures in which foreigners are occasionally encountered. In the model three parameters are used to represent an open social structure; group size, memory length and the starting proportion of xenophobes. These parameters determine how often "foreigners" will be encountered and whether or not people will change their behaviour towards foreigners. These parameters together with the length of time (number of interactions) before which a strategy change can be made and a simple tit-for-tat strategy provide us with the most necessary tools for a modelling of the question. But despite the simplicity of this model the answer to the question proves somewhat more complex than expected: -

• A high degree of cooperation and a low level of xenophobia can be established through evolution. But only if:
  • changes in behaviour don't take place too often (approximate memory length)
  • the starting xenophobe and co-operator proportions are not equally large.
• If these conditions are not met the long-term developments in the system will be self-organized and unpredictable. In this case it is also possible that unstable developments will take place and the proportion of xenophobes will fluctuate constantly.
• In smaller groups stable relations are established more quickly and more reliably, but
  • the starting proportion of xenophobes is usually not reduced. Instead, a high proportion of xenophobes is often retained.
  • if a certain level of experience has been reached and the number of xenophobes is not very large, xenophobes have no further reason to change their behaviour; they remain xenophobic.

5.2

On the one hand, the results of this minimally constructed model give us reason to hope that even in open social structures with quite typical social conditions the amount of cooperative behaviour will increase through evolution. On the other hand, this model makes it clear that belief in individual rationality alone is not sufficient for the establishment of a high degree of cooperation and the prevention of instability. Furthermore the results of this model legitimise the continued existence of political institutions that ensure cooperation.

Notes

¹ Many of the results in Coleman (1992) were presented in Coleman (1986). But in Coleman (1992) the more interesting section that includes variable strategies (see section 4) was expanded.

² For other reasons why a "demand for norms" comes into being when a decision must be made to cooperate or defect see Coleman (1986, 1992, 1990: chap. 10).

³"Suppose further that each animal can recognize individuals it has already interacted with and can remember salient aspects of their interaction, such as whether the other has usually cooperated" (Axelrod 1984:49)

⁴"So long as the expected time to the next interaction with a particular other is far into the future (as it will be in large populations Axelrod envisions), and so long as there is discounting of the future payoffs, a defect strategy will escape sanctions and will dominate all others." (Coleman 1986:66)

⁵ I hope to have thus acted on Coleman's observation that "Further work on the emergence of norms clearly appears profitable" (Coleman 1986:83).

⁶ For a discussion of the theoretical implications of this modelling (e.g. Axelrod and Hamilton 1981, Maynard Smith and Price 1973) consider the corresponding sections in Coleman (1992, 1990).

⁷ For a discussion of the advantages and disadvantages of using this game to model the evolution of cooperation see Coleman 1992:254f. Because of its simplicity (the exclusion of externalities which affect third parties and the fact that there are only two possible manners of behaviour) it is most certainly "a useful starting point" (256).

⁸ The group size N is based on the idea of "neighbourhood" and "embededness" in other models (Macy and Skvoretz 1998:655)

⁹ The memory length can thus be compared with Axelrod's "shadow of the future" and the accompanying discount-Parameter alfa. Hegselmann refers to (1-alfa) as the "degree of anonymity" (anonymisierungsgrad) in the society (Hegselmann 1992:187). The smaller the alfa and the shadow of the future, the more likely it is that the next interaction will be with a "foreigner". Memory length also plays a role in strategy-models based on genetic algorithms. When, for example, one can remember an opponent's last three moves, there are 2¹⁵ possible strategies (Lomborg 1996:284). But in Lomborg's model all potential opponents are recognized, a fact which contradicts the intuitively limited nature of memory.

¹⁰ Another way of allowing the social structure to have an effect would be to use a model in which the division of the social structure is already set - a closely connected network (honeycombs) of neighbours for example (Axelrod 1984, Hegselmann 1996, Liebrand and Messick 1996).

¹¹ Axelrod refers to this move as "contrition" (Axelrod 1997:37). Especially when noise is present, this strategy is advantageous. The effect of noise is comparable to the presence of foreigners.

¹² This is of course only the description of a minimal strategy and other strategies, especially long-term strategies, are also plausible. But this minimal strategy is nonetheless intuitively plausible and, as far as profits are concerned, Axelrod's results demonstrate that this strategy is ideal in the long-term.

¹³The simulation program and its description are available at the author's internet-site: http://mzs.sowi.uni-goettingen.de/mitarbeitende/mueller-benedict/benedict.shtml or http://mzs.sowi.uni-goettingen.de/downloads/software/software.shtml

¹⁴ In this phase of the fixed strategy scenario the exact values of the payoffs in a prisoner's-dilemma situation are still unimportant; it is only important to know that cooperation leads to the highest payoff values.

¹⁵ Coleman (1990: 241f., 325f.) discusses a number of ways that sanctions can be established in order to maintain cooperation.

¹⁶ See as well figures 4.1 - 4.5 in Coleman ( 1986:76-80)

¹⁷ In 1986 Coleman concludes: "it turns out that the evolving system and the non-evolving system are scarcely distinguishable". In 1992 he presents an example calculation in which he assesses the long-term payoffs of all possible strategies when the group size is four. This calculation shows that it is plausible that the developments in this system will tend towards cooperation in the long run. But this assessment was only made for a single group size.

¹⁸ The nominator is the inverse of the payoff value and the denominator is the number of meetings in this round. The total number of meetings is the sum of all denominators. But, because of the randomly generated pairings of people, in which people sometimes meet themselves (encounters which do not count), this value doesn't equal 60.

¹⁹ In this paper a system will be defined as "self-organized" when the long-term end-state is unpredictable and is not determined by starting conditions. Such systems are, for example, chaotic systems or systems that have bifurcation points.

References

AXELROD, ROBERT and HAMILTON, W.D. 1981: The Evolution of cooperation. Science 211:1390-96

AXELROD, ROBERT 1984: The Evolution of Cooperation. New York: Basic Books

AXELROD, ROBERT 1997: The complexity of cooperation: agent-based models of competition an cooperation. Princeton: University Press

COLEMAN, JAMES S. 1986: Social Structure and the Emergence of Norms among Rational Actors. p. 55-85 in: DIEKMANN, ANDREAS and MITTER, PETER: Paradoxical Effects of Social Behaviour. Essays in Honor of Anatol Rapoport. Heidelberg:Physica 1986

COLEMAN, JAMES S. 1990: Foundations of Social Theory. Belknapp

COLEMAN, JAMES S. 1992: Norm-Generating Structures. p. 250-273 in: COOK, KAREN S. and LEVI, MARGRET: The Limits of Rationality. Chicago:University Press 1992

HAUSKEN, K. 2000: Cooperation and between-group competition. Journal of Economic Behaviour & Organization 42:417-425

HEGSELMANN, RAINER 1996: Cellular Automata in the Social Sciences. Perspectives, Restrictions, Artefacts. P. 209-234 in: HEGSELMANN, RAINER, Mueller, ULRICH and TROTZSCH, KLAUS G: (eds.): Modelling and Simulation in he Social Sciences from the Philosophy of Science Point of View. Dordrecht:Kluwer 1996

HEGSELMANN, RAINER 1992: Moralität im iterierten Gefangenen-Dilemma. p. 183-190 in HOLLIS, MARTIN and VOSSENKUHL, WILHELM (eds.): Moralische Entscheidung und rationale Wahl. München:Oldenbourg

HOBBES, THOMAS 1950 [1651]: Leviathan. New York: Dutton

HOFFMANN, R. 2000: Twenty Years on: The Evoluation of Cooperation Revisited. Journal of Artificial Societies and Social Simulation, vol 3, no. 2 <http://www.soc.sourrey.ac.uk/JASSS/3/2/forum/1.html >

LIEBRAND, W. and MESSNICK, DAVID: Computer Simulations of sustainable cooperation in social Dilemmas. p. 235-248 in: HEGSELMANN, RAINER, Mueller, ULRICH and TROTZSCH, KLAUS G: (eds.): Modelling and Simulation in he Social Sciences from the Philosophy of Science Point of View. Dordrecht:Kluwer 1996

LOMBORG,, B. 1996: Nucleus and Shield: The Evolution of Social Structure in the Iterated Prisoner's Dilemma. American Sociological Review 61:287-301

MACY, M., SKVORETZ, J. 1998: The Evolution of Trust and Cooperation Between Strangers: A Computational Model. American Sociological Review 63:638-660

MAYNARD SMITH, JOHN and PRICE, G. R. 1973: The logic of animal conflict. Nature 246:15-18

ORBELL, J., ZENG, L., MULFORD, M. 1996: Individual Experience and The Fragmentations od Societies. American Sociological Review 61:1018-1032

RAPOPORT, ANATOL and CHAMMAH, ALBERT M 1965: Prisoners Dilemma. Ann Arbor:University Press

ROUSSEAU, JACQUES 1950 [1756]: The Social Contract. New York:Dutton

VOGT, C. 2000: The Evolution of Cooperation in Prisoner's Dilemma with an Endogenous Learning Mutant. Journal of Economic Behaviour & Organization 42:347-373

WEBER, MAX 1976 [1925] : Wirtschaft und Gesellschaft . Tübingen:Mohr

Return to Contents of this issue

© Copyright Journal of Artificial Societies and Social Simulation, [2002]