Stephen Younger (2005)
Reciprocity, Sanctions, and the Development of Mutual Obligation in Egalitarian Societies
Journal of Artificial Societies and Social Simulation
vol. 8, no. 2
<https://www.jasss.org/8/2/9.html>
To cite articles published in the Journal of Artificial Societies and Social Simulation, reference the above information and include paragraph numbers if necessary
Received: 26-Oct-2004 Accepted: 17-Dec-2004 Published: 31-Mar-2005
 Abstract
Abstract| Table 1: Effect of different models of sharing on the total mutual obligation within the artificial society | ||
| Model for Sharing | Mutual Obligation | Standard Deviation |
| Indiscriminant Sharing | 330 | 58 |
| Share Only with Head of Household | 160 | 21 |
| Share Only Within Family | 190 | 21 |
| Share Only With Other Sharing Agents* | 210 | 20 |
| * Only six of the twenty runs in this case had a population that survived until the end of the run. All other models, had a non-zero population at the end of every run. | ||
| Table 2: Comparison of the simulation results with the observations of the Mer Islanders. The first three columns are taken from Bliege Bird and Bird (1997) | |||||
| Observations of the Mer Islanders | Simulation | ||||
| Model | Prediction | Result | Rule | Result | |
| Kinship | Households with no patrilineal kin nearby should send shares to kin living farther away rather than share with nonkin neighbors | Not supported | Share only within family | Mutual obligation lower than for indiscriminant sharing | |
| Tolerated Theft | Larger households should receive larger portions of turtle meat from the distributing household at each butchery event | Supported | Share with head of household only independent of size of family | Mutual obligation lower than for sharing with each individual | |
| Risk-reduction Reciprocity | Households that never hunt should never receive shares | Not supported | Do not share with non-sharing agents | Mutual obligation lower than for indiscriminant sharing | |

|
| Figure 1. Percentage of runs where one, both, or neither subpopulation of normative and non-normative agents survive until the end of the run. Note that for toltheft = -1000000, no sanctions were ever applied to non-normative agents, so we present only the results of the Baseline and Transfer scenarios |
|
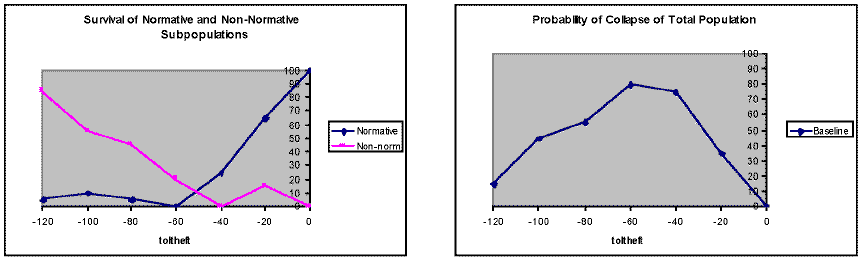
| Figure 2. Left: Probability of survival for normative and non-normative subpopulations as a function of toltheft, the tolerance to theft. Right: Percent probability of collapse for the total population. Here toltheft = 0 implies zero tolerance to theft and toltheft = -120 implies a large tolerance to theft. All curves were computed for the Baseline scenario |
|
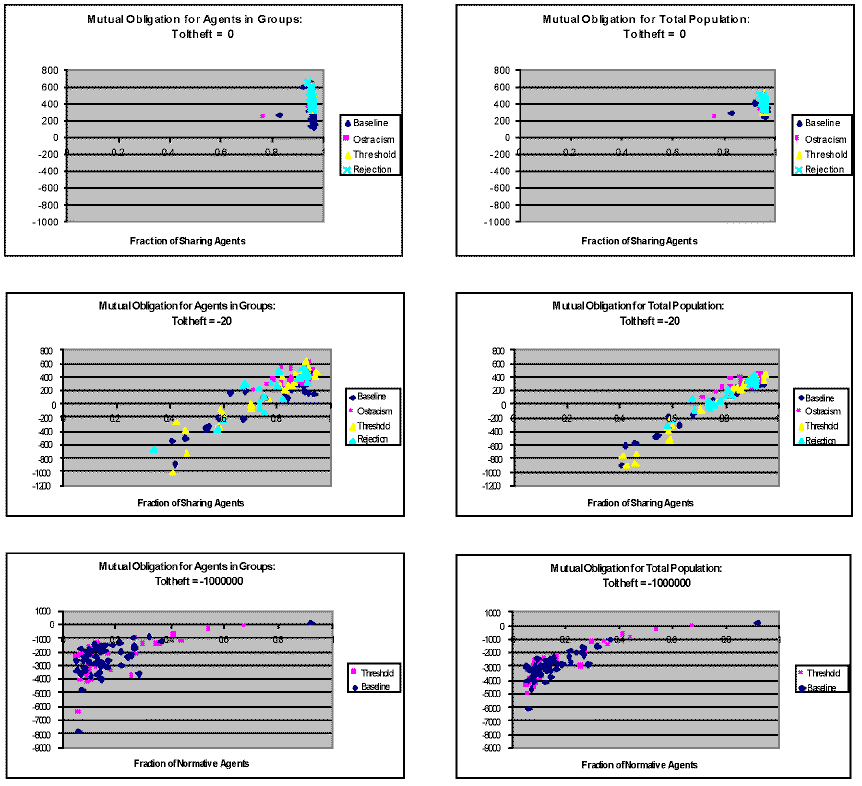
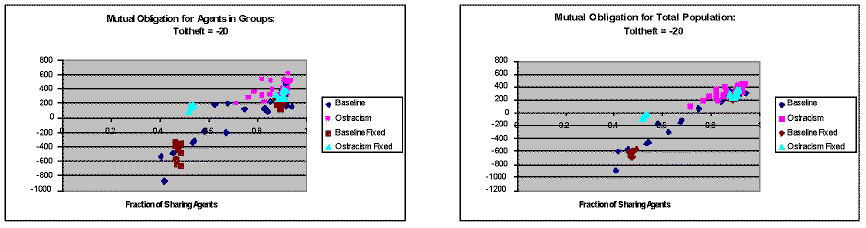
| Figure 3. Mutual obligation factors for the total population and for agents in groups. Mutual obligation was defined as the sum of the interaction matrix elements connecting agents in the population. Sharing contributed to, and stealing detracted from, the interaction matrix elements |
| Table 3: Averages and standard deviations for key parameters taken over all runs that had a non-zero population at the end of the run | |||||||||||||
| Tolerance = 0 | Tolerance = -20 | Tolerance = -1,000,000 | |||||||||||
| Baseline | Transfer | Rejection | Ostracism | Baseline | Transfer | Rejection | Ostracism | Baseline | Transfer | ||||
| Fraction Normative | 0.96 | 0.96 | 0.95 | 0.95 | 0.76 | 0.74 | 0.78 | 0.88 | 0.16 | 0.16 | |||
| Standard Deviation | 0.0038 | 0.0031 | 0.0044 | 0.028 | 0.18 | 0.18 | 0.14 | 0.054 | 0.13 | 0.13 | |||
| Mutual Obligation | |||||||||||||
| Normative Agents | 350 | 400 | 410 | 400 | 370 | 400 | 400 | 400 | 130 | 120 | |||
| Standard Deviation | 50 | 61 | 51 | 47 | 73 | 73 | 76 | 65 | 57 | 66 | |||
| Non-Normative Agents | -140 | -140 | -140 | -130 | -810 | -910 | -820 | -260 | -3400 | -3400 | |||
| Standard Deviation | 16 | 22 | 18 | 17 | 400 | 490 | 360 | 84 | 920 | 1000 | |||
| Total Population | 330 | 370 | 390 | 370 | 37 | -5.7 | 96 | 310 | -2900 | -2900 | |||
| Standard Deviation | 48 | 58 | 48 | 48 | 380 | 440 | 360 | 84 | 990 | 1120 | |||
| Agents in Groups | 300 | 400 | 430 | 400 | 79 | 110 | 220 | 380 | -2400 | -2500 | |||
| Standard Deviation | 85 | 75 | 74 | 62 | 350 | 410 | 300 | 98 | 1200 | 1200 | |||
| Sharing Rate per Agent | 0.045 | 0.051 | 0.050 | 0.050 | 0.038 | 0.039 | 0.040 | 0.049 | 0.0087 | 0.0091 | |||
| Standard Deviation | 0.0053 | 0.0030 | 0.0038 | 0.0061 | 0.010 | 0.0089 | 0.0066 | 0.0039 | 0.0055 | 0.0075 | |||
| Stealing Rate per Agent | 0.039 | 0.039 | 0.039 | 0.037 | 0.020 | 0.023 | 0.019 | 0.011 | 0.044 | 0.045 | |||
| Standard Deviation | 0.00040 | 0.00042 | 0.00043 | 0.00045 | 0.012 | 0.015 | 0.012 | 0.0059 | 0.0061 | 0.0043 | |||
| Percent Runs that Survive | 94 | 92 | 92 | 96 | 50 | 54 | 44 | 64 | 100 | 96 | |||
|
| Figure 4. Comparison of the mutual obligation for the total population and for agents in groups for the case of matrilineal inheritance of normative character and for a fixed distribution of 50% and 90% sharing agents. Mutual obligation was defined as the sum of the interaction matrix elements connecting agents in the population. Sharing contributed to, and stealing detracted from, the interaction matrix elements |
BLIEGE BIRD RL and Bird, DW (1997) "Delayed Reciprocity and Tolerated Theft: The Behavioral Ecology of Food-Sharing Strategies", Current Anthropology 38, 49-78.
BLURTON JONES, NG (1984) "A Selfish Origin for Human Food Sharing: Tolerated Theft", Ethology and Sociobology 5, 1-3.
BOEHM C (1999) Hierarchy in the Forest: The Evolution of Egalitarian Behavior (Harvard University Press, Cambridge).
CASTELFRANCHI C, Conte R, and Paolucci M (1998) "Normative Reputation and the Costs of Compliance", Journal of Artificial Societies and Social Simulation 1 (3).
HALES D (2002) "Group Reputation Supports Beneficent Norms", Journal of Artificial Societies and Social Simulation 5 (4).
GURVEN M, Allen-Arave W, Hill K, and Hurtado M, (2000) "It's a Wonderful Life: Signaling Generosity Among the Ache of Paraguay", Evolution and Human Behavior 21, 263-282.
GURVEN M (2003) "To Give or Give Not: The Behavioral Ecology of Human Food Transfers", Behavioral and Brain Sciences
HILL K (2004) Private Communication.
JAFFE K (2002) "An Economic Analysis of Altruism: Who Benefits from Altruistic Acts?" Journal of Artificial Societies and Social Simulation 5 (3).
KAPLAN H and HILL K (1985) "Food Sharing Among Ache Foragers: Tests of Explanatory Hypotheses", Current Anthropology 26, 223-246.
KENT S (1993) "Sharing in an Egalitarian Community", Man, 28, 479-514.
MAUSS M (1990[1950]) The Gift: The Form and Reason for Exchange in Archaic Societies (Norton, New York).
PETERSON N (1993) "Demand Sharing: Reciprocity and the Pressure for Generosity among Foragers", American Anthropologist, New Series 95, 860-874.
WOODBURN J (1982) "Egalitarian Societies", Man 17, 431-451.
YOUNGER S M (2003) "Discrete Agent Simulations of the Effect of Simple Social Structures on the Benefits of Resource Sharing", Journal of Artificial Societies and Social Simulation 6 (3).
YOUNGER SM (2004) "Reciprocity, Normative Reputation, and the Development of Mutual Obligation in Gift-Giving Societies", Journal of Artificial Societies and Social Simulation, 7(1).
YOUNGER SM (2004b) "Reciprocity, Tolerated Theft, and Reproduction Strategies in Foraging Societies", Los Alamos National Laboratory Report LA-UR-04-7119.
Return to Contents of this issue
© Copyright Journal of Artificial Societies and Social Simulation, [2005]